Are you looking for an answer to the topic “Transient time response of a Second order (Underdamped) System with 0 δ(damping ratio) 1“? We answer all your questions at the website Chambazone.com in category: 40+ Marketing Blog Topics & Ideas. You will find the answer right below.
Keep Reading
Intro to Control – 9.3 Second Order System: Damping \u0026 Natural Frequency
Images related to the topicIntro to Control – 9.3 Second Order System: Damping \u0026 Natural Frequency
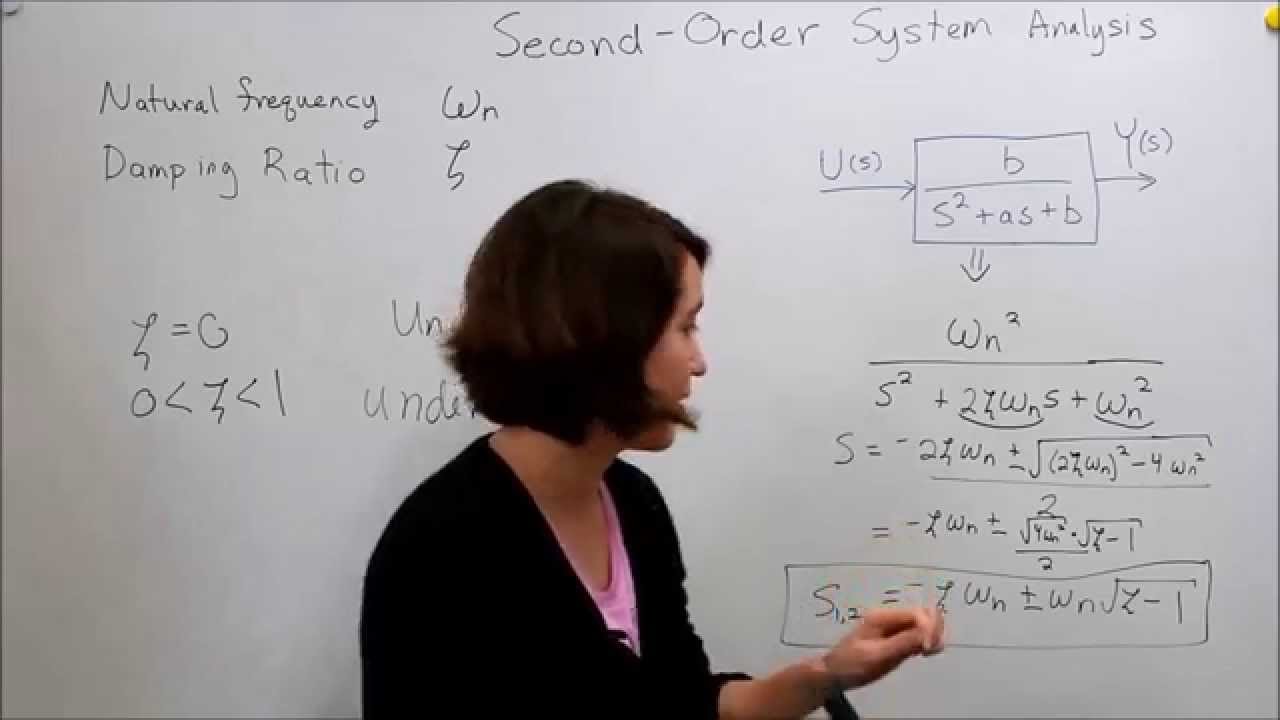
Spoiler: This article will be a little hard to understand at first. I invite you to also read some standard books as the derivation part for reference. Finally, please be patient and enjoy the post! In my previous post, we saw the time response of a first-order system as well as a second-order general closed-loop transfer function. In this article, we will now deal with the temporal behavior of second-order systems (mostly underdamped systems). Before that, let’s summarize the important types we covered in the previous article, the different types of systems (based on the value of the “damping factor”). You can refer to the graph below: In the graph above, the damping ratio ( ) is represented as . In all four decay cases, the most important is when 0 < < 1, which is H. "Underdamped" system type. We must be very aware of "underdamped systems". We now examine the behavior of an underdamped second-order system with unit momentum and unit step input. Derivation of the Unity Impulse Response for a Second-Order "Undamped" System: The standard second-order transfer function is given by: The poles can be obtained by setting the denominator term to zero. The poles can be given by assuming, then we have, now conser an underdamped system (< 1). If < 1, we have complex zeros. R(s) is also 1 because we are consering a "pulse input" unit. (setting R(s)=1) The above equation can be rearranged as, multiplying and diving by '' we get, but therefore, to get the equation for c(t), we need to calculate the Laplace inverse of C(s), We already know (from the inverse Laplace theory), therefore, that, in this case, the system is called an underdamped system. Unit Step Response Derivation for an "Undamped" Second-Order System: Let us now obtain the response c(t) to a unit step input R(s). We know that for a unit step input, R(s) = 1/s so the underdamped case (0 < δ < 1) : : (by setting R(s) = 1/s for the step input) This can be done by using Solve some fractions. As in (0 < 1), the roots of the second-order transfer function are complex numbers. Also, we will make some adjustments to make the equation easier to solve. Among them, "" is called the damped natural frequency, and "" is called the undamped natural frequency. We can now use the Laplace transform and get something like this cos α = sin α = we have sinA cosB + cosA sinB = sin (A + B) Substituting in the desired value we get c(t) = 1 – sin ( t + α ) and tan α = i.e. α = c(t) = 1 – sin ( t + ) , which represents the solution for 0 < < 1 under damping. We can see that the response contains a sine term multiplied by an exponential decay term. So the end result is a sinusoal decay on an exponential envelope. Some points to note from the adjacent graphs (see both graphs): 1. We can see that the response crosses the reference and oscillates before settling to its final value. Therefore underdamped systems exhibit overshoot and undershoot. 2. The poles are in quadrants 2 and 3. The real part of the pole also produces a decaying exponential function, while the imaginary part produces a sine function. Before I end my post here, I want to give you one last thought, as we've seen response characteristics including overshoot and undershoot (also sometimes based on pre-settling delays). So there must be some well-defined parameters to understand the full behavior of the response. wrong? ? … (See full instructions in my next post) Report this ad
See some more details on the topic Transient time response of a Second order (Underdamped) System with 0 δ(damping ratio) 1 here:
Transient time response of a Second order (Underdamped …
Control System – Transient time response of a Second order (Underdamped) System with 0 < δ(damping ratio) < 1 · Spoiler : This post will be a little difficult to ...
The transient response of second order under damped system …
Second-order underdamped system: Transient response of second-order … Damping ratio Response 1 δ = 0 Critically damped 2 0 < δ < 1 Under damped 3 δ = 1 ...
Time Response of Second Order Control System (Worked …
There will be different behaviors of output signal, depending upon the value of damping ratio and let us examine each of the cases, one by one.
Underdamped System – an overview | ScienceDirect Topics
In the undamped system that we consered first, also Δ < 0, and the system ... When the damping ratio is between 0 and 1 (i.e. underdamped system), ...
Related searches to Transient time response of a Second order (Underdamped) System with 0 δ(damping ratio) 1
- first order system
- steady state error
- find the transfer function from the differential equations
- settling time of second order system
- Steady state error
- First order system
- peak time in control system
- Step response of second order system
- what is the difference between 1st order and 2nd order models
- step response of second order system
- name three performance specification for a second order underdamped system
- Settling time of second order system
- Find the transfer function from the differential equations
- Peak time in control system
Information related to the topic Transient time response of a Second order (Underdamped) System with 0 δ(damping ratio) 1
Here are the search results of the thread Transient time response of a Second order (Underdamped) System with 0 δ(damping ratio) 1 from Bing. You can read more if you want.
You have just come across an article on the topic Transient time response of a Second order (Underdamped) System with 0 δ(damping ratio) 1. If you found this article useful, please share it. Thank you very much.
